Anmelden (DTAQ) DWDS     dlexDB     CLARIN-D

Wolff, Christian von: Der Anfangs-Gründe Aller Mathematischen Wiessenschaften. Bd. 4. Halle (Saale), 1710.

Bild:
<< vorherige Seite
nächste Seite >>

der Algebra.
x3 + 4cx2 + 4c2x-4abc = o.
- 2abx + 2a2d
+ a2x

Hieraus sehet ihr/ daß/ wenn die wahre Wurtzel zur
Rechten fallen sol/ das andere Glied das Zeichen +
haben muß und/ wenn c fehlet/ der Punct A in a
fället. Damit ihr nun auch die Werthe von c/ b
und d findet/ die in der Central-Regel angegeben
worden; so setzet
x3 + px2 + qx + r = o.
Dann ist
4c = p 4c2 - 2ab + a2 = q
c = 1/4p 4c2 + a2 - q = 2ab
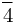p2 + a2 - q = 2ab
2a
1/2a + p2 :8a - q : 2a = b
a2 + 4c2 - 2ab = -q
a2 + 1/4p2 + q = 2ab
2a
1/2a + p2 : 8a + q : 2a = b
2a2d - 4abc = r
2a2d = 4abc + r
d = 2bc:a + r : 2a2
das ist/ d = 1/4p + p3 : 16a2 + pq : 4a2 + r : 2a2
2a2d - 4abc = - r

2a2
P 5

der Algebra.
x3 + 4cx2 + 4c2x-4abc = o.
- 2abx + 2a2d
+ a2x

Hieraus ſehet ihr/ daß/ wenn die wahre Wurtzel zur
Rechten fallen ſol/ das andere Glied das Zeichen +
haben muß und/ wenn c fehlet/ der Punct A in a
faͤllet. Damit ihr nun auch die Werthe von c/ b
und d findet/ die in der Central-Regel angegeben
worden; ſo ſetzet
x3 + px2 + qx + r = o.
Dann iſt
4c = p 4c2 - 2ab + a2 = q
c = ¼p 4c2 + a2 - q = 2ab
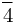p2 + a2 - q = 2ab
2a
½a + p2 :8a - q : 2a = b
a2 + 4c2 - 2ab = -q
a2 + ¼p2 + q = 2ab
2a
½a + p2 : 8a + q : 2a = b
2a2d - 4abc = r
2a2d = 4abc + r
d = 2bc:a + r : 2a2
das iſt/ d = ¼p + p3 : 16a2 + pq : 4a2 + r : 2a2
2a2d - 4abc = - r

2a2
P 5
<TEI>
  <text>
    <body>
      <div n="1">
        <div n="2">
          <div n="3">
            <div n="4">
              <div n="5">
                <p>
                  <pb facs="#f0235" n="233"/>
                  <fw place="top" type="header"> <hi rendition="#b">der Algebra.</hi> </fw><lb/> <hi rendition="#et"> <hi rendition="#aq"><hi rendition="#i">x</hi><hi rendition="#sup">3</hi> + 4<hi rendition="#i">cx</hi><hi rendition="#sup">2</hi> + 4<hi rendition="#i">c</hi><hi rendition="#sup">2</hi><hi rendition="#i">x</hi>-4<hi rendition="#i">abc = o.</hi><lb/>
- 2<hi rendition="#i">abx</hi> + 2<hi rendition="#i">a</hi><hi rendition="#sup">2</hi><hi rendition="#i">d<lb/>
+ a</hi><hi rendition="#sup">2</hi><hi rendition="#i">x</hi></hi> </hi> </p><lb/>
                <p>Hieraus &#x017F;ehet ihr/ daß/ wenn die wahre Wurtzel zur<lb/>
Rechten fallen &#x017F;ol/ das andere Glied das Zeichen +<lb/>
haben muß und/ wenn <hi rendition="#aq"><hi rendition="#i">c</hi></hi> fehlet/ der Punct <hi rendition="#aq">A</hi> in <hi rendition="#aq"><hi rendition="#i">a</hi></hi><lb/>
fa&#x0364;llet. Damit ihr nun auch die Werthe von <hi rendition="#aq"><hi rendition="#i">c/ b</hi></hi><lb/>
und <hi rendition="#aq"><hi rendition="#i">d</hi></hi> findet/ die in der Central-Regel angegeben<lb/>
worden; &#x017F;o &#x017F;etzet<lb/><hi rendition="#et"><hi rendition="#aq"><hi rendition="#i">x</hi><hi rendition="#sup">3</hi> + <hi rendition="#i">px</hi><hi rendition="#sup">2</hi> <hi rendition="#u">+</hi> <hi rendition="#i">qx</hi> <hi rendition="#u">+</hi> <hi rendition="#i">r</hi> = <hi rendition="#i">o.</hi></hi></hi><lb/>
Dann i&#x017F;t<lb/><hi rendition="#et"><hi rendition="#aq"><hi rendition="#u">4<hi rendition="#i">c = p</hi> 4<hi rendition="#i">c</hi><hi rendition="#sup">2</hi> - 2<hi rendition="#i">ab + a</hi><hi rendition="#sup">2</hi> = <hi rendition="#i">q</hi></hi><lb/><hi rendition="#i">c</hi> = ¼<hi rendition="#i">p</hi> <hi rendition="#u">4<hi rendition="#i">c</hi><hi rendition="#sup">2</hi> + <hi rendition="#i">a</hi><hi rendition="#sup">2</hi> - <hi rendition="#i">q</hi> = 2<hi rendition="#i">ab</hi><lb/><formula notation="TeX">\frac {}{4}</formula><hi rendition="#i">p</hi><hi rendition="#sup">2</hi> + <hi rendition="#i">a</hi><hi rendition="#sup">2</hi> - <hi rendition="#i">q</hi> = 2<hi rendition="#i">ab</hi></hi><lb/>
2<hi rendition="#i">a</hi><lb/>
½<hi rendition="#i">a + p</hi><hi rendition="#sup">2</hi> :8<hi rendition="#i">a - q</hi> : 2<hi rendition="#i">a = b</hi><lb/><hi rendition="#u"><hi rendition="#i">a</hi><hi rendition="#sup">2</hi> + 4<hi rendition="#i">c</hi><hi rendition="#sup">2</hi> - 2<hi rendition="#i">ab = -q<lb/>
a</hi><hi rendition="#sup">2</hi> + ¼<hi rendition="#i">p</hi><hi rendition="#sup">2</hi> + <hi rendition="#i">q</hi> = 2<hi rendition="#i">ab</hi></hi><lb/>
2<hi rendition="#i">a</hi><lb/>
½<hi rendition="#i">a + p</hi><hi rendition="#sup">2</hi> : 8<hi rendition="#i">a + q : 2a = b</hi><lb/><hi rendition="#u">2<hi rendition="#i">a</hi><hi rendition="#sup">2</hi><hi rendition="#i">d</hi> - 4<hi rendition="#i">abc = r</hi><lb/>
2<hi rendition="#i">a</hi><hi rendition="#sup">2</hi><hi rendition="#i">d</hi> = 4<hi rendition="#i">abc + r</hi></hi><lb/><hi rendition="#i">d</hi> = 2<hi rendition="#i">bc:a + r</hi> : 2<hi rendition="#i">a</hi><hi rendition="#sup">2</hi></hi></hi><lb/>
das i&#x017F;t/ <hi rendition="#aq"><hi rendition="#i">d</hi> = ¼<hi rendition="#i">p + p</hi><hi rendition="#sup">3</hi> : 16<hi rendition="#i">a</hi><hi rendition="#sup">2</hi> + <hi rendition="#i">pq</hi> : 4<hi rendition="#i">a</hi><hi rendition="#sup">2</hi> + <hi rendition="#i">r</hi> : 2<hi rendition="#i">a</hi><hi rendition="#sup">2</hi><lb/><hi rendition="#et"><hi rendition="#u">2<hi rendition="#i">a</hi><hi rendition="#sup">2</hi><hi rendition="#i">d</hi> - 4<hi rendition="#i">abc = - r</hi></hi></hi></hi><lb/>
<fw place="bottom" type="sig">P 5</fw><fw place="bottom" type="catch">2<hi rendition="#aq"><hi rendition="#i">a</hi></hi><hi rendition="#sup">2</hi></fw><lb/></p>
              </div>
            </div>
          </div>
        </div>
      </div>
    </body>
  </text>
</TEI>
[233/0235] der Algebra. x3 + 4cx2 + 4c2x-4abc = o. - 2abx + 2a2d + a2x Hieraus ſehet ihr/ daß/ wenn die wahre Wurtzel zur Rechten fallen ſol/ das andere Glied das Zeichen + haben muß und/ wenn c fehlet/ der Punct A in a faͤllet. Damit ihr nun auch die Werthe von c/ b und d findet/ die in der Central-Regel angegeben worden; ſo ſetzet x3 + px2 + qx + r = o. Dann iſt 4c = p 4c2 - 2ab + a2 = q c = ¼p 4c2 + a2 - q = 2ab [FORMEL]p2 + a2 - q = 2ab 2a ½a + p2 :8a - q : 2a = b a2 + 4c2 - 2ab = -q a2 + ¼p2 + q = 2ab 2a ½a + p2 : 8a + q : 2a = b 2a2d - 4abc = r 2a2d = 4abc + r d = 2bc:a + r : 2a2 das iſt/ d = ¼p + p3 : 16a2 + pq : 4a2 + r : 2a2 2a2d - 4abc = - r 2a2 P 5

Suche im Werk

Hilfe

Informationen zum Werk

Download dieses Werks

XML (TEI P5) · HTML · Text
TCF (text annotation layer)
TCF (tokenisiert, serialisiert, lemmatisiert, normalisiert)
XML (TEI P5 inkl. att.linguistic)

Metadaten zum Werk

TEI-Header · CMDI · Dublin Core

Ansichten dieser Seite

Voyant Tools ?

Language Resource Switchboard?

Feedback

Sie haben einen Fehler gefunden? Dann können Sie diesen über unsere Qualitätssicherungsplattform DTAQ melden.

Kommentar zur DTA-Ausgabe

Dieses Werk wurde gemäß den DTA-Transkriptionsrichtlinien im Double-Keying-Verfahren von Nicht-Muttersprachlern erfasst und in XML/TEI P5 nach DTA-Basisformat kodiert.




Ansicht auf Standard zurückstellen

URL zu diesem Werk: https://www.deutschestextarchiv.de/wolff_anfangsgruende04_1710
URL zu dieser Seite: https://www.deutschestextarchiv.de/wolff_anfangsgruende04_1710/235
Zitationshilfe: Wolff, Christian von: Der Anfangs-Gründe Aller Mathematischen Wiessenschaften. Bd. 4. Halle (Saale), 1710. , S. 233. In: Deutsches Textarchiv <https://www.deutschestextarchiv.de/wolff_anfangsgruende04_1710/235>, abgerufen am 16.05.2024.